Multiple Beam Interferometry
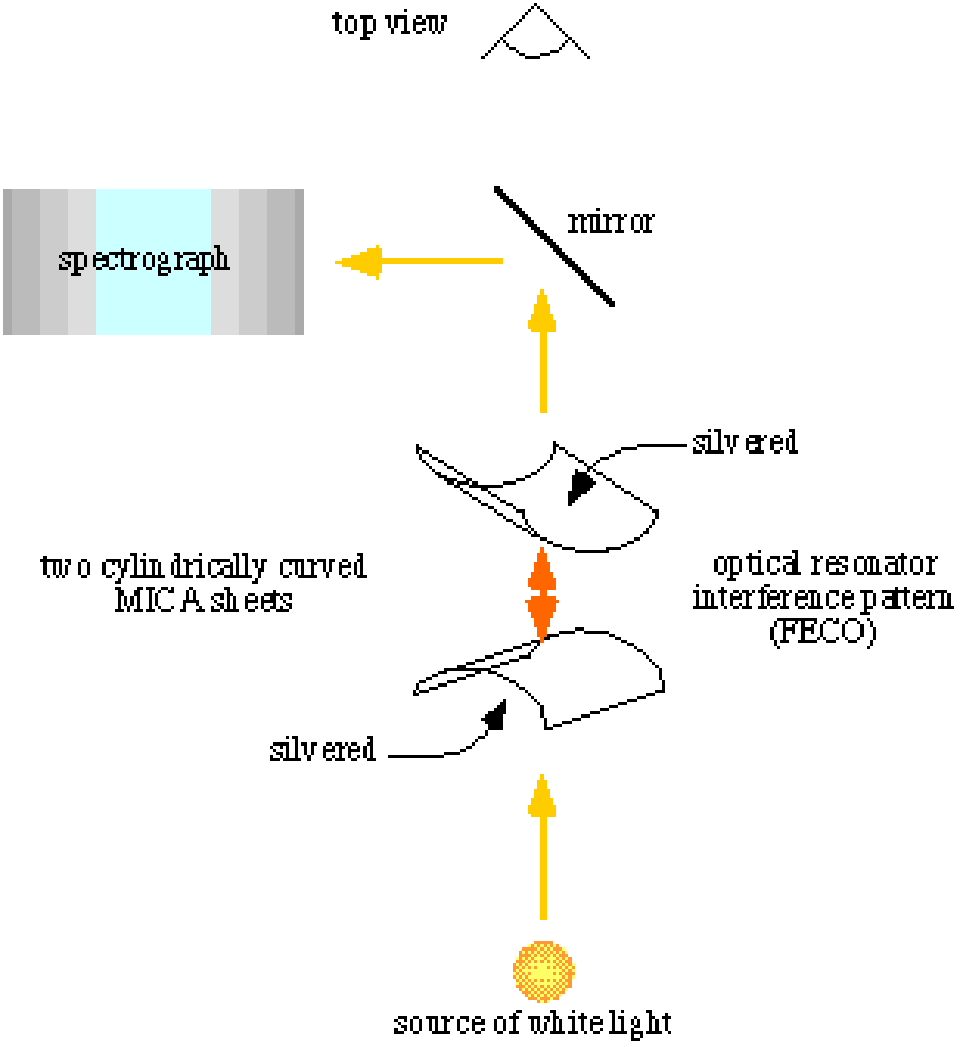
Multiple Beam Interferometry (MBI) is used in the Surface Forces Apparatus (SFA) to measure the distance between the two surfaces of interest with high accuracy. Interferometry allows one to measure distances down to 1/1000 of a wavelength. MBI uses intense white light which is sent normally through the surfaces in the SFA. Each surface (usually MICA) has a highly reflecting silver coating on one side which acts as a mirror. The white light is reflected multiple times on these mirrors before it leaves the interferometer, each time interfering with the previously reflected beams. Some particular wavelengths fit exactly an entire number of times inside the interferometer and lead to constructive interference. Almost like the simple example of a standing wave along a guitar string. However, the electric field inside the mirrors actually is not a standing wave. Since there is transport of net energy, nodes as well as antinodes propagate in the direction of the energyflow.
MBI is sensitive to the refractive index in the space between the mirrors. Hence, MBI can be very useful for experiments involving a huge variety of molecules between the surfaces.
The Figure 1 shows a schematic MBI setup used in the SFA. A source of white light is focussed between two silver-coated surfaces. The light emerging from the interferometer consists of well defined wavelengths which fit exactly an entire number of times into the optical resonnator.
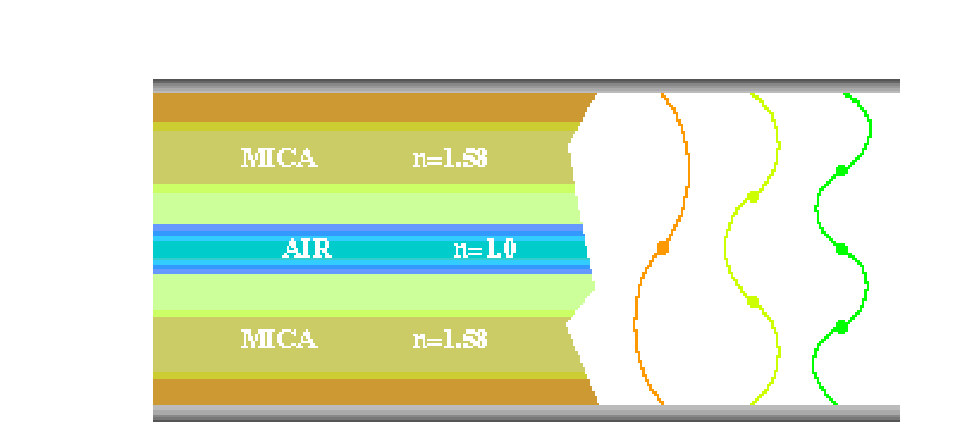
The Figure 2 displays a crossection through an interferometer which is made of two paralel MICA-layers and an air gap between them. To the right, the electric field distribution for the first three constructively interfering beams that form a fringe are shown schematically. At any given time, the electric field of a given fringe exhibits a number of nodes which determines its chromatic order.
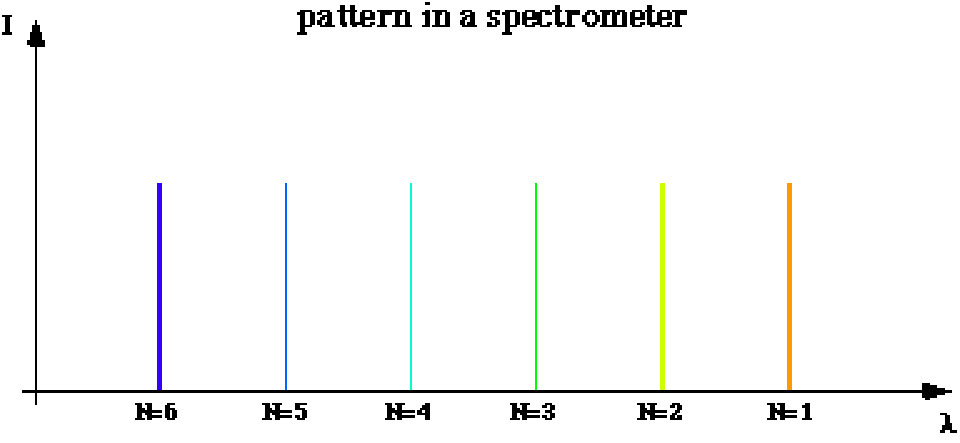
As shown below, one can observe a sharp intensity peak corresponding to each chromatic order N. The wavelength difference between the fringes is a measure for thethicknes and refractive index of the layers inside the mirrors.
The Figure 3 below shows the appearance of a fringe pattern in a spectrometer. Each fringe corresponds to an entire number of wavelengths fitting exactly inside the mirrors.
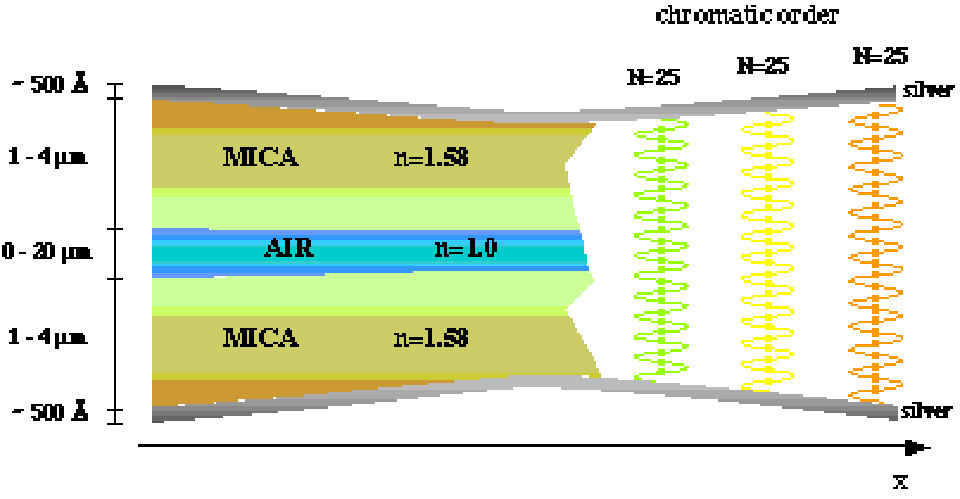
In a real SFA/MBI experiment, the surfaces are curvedaccording to the shape of a cylinder, so that the distance between the silver mirrors becomes a function of the lateral position. If one considers a fringe of order say N=25 (figure below), then it is clear that this fringe will undergo a wavelength-shift to longer wavelengths the further out we go laterally. This is why fringes reflect the local geometry of the surfaces in the experiment. The corresponding electric field is sketched in the following Figure 4.
In complete analogy, all the other fringes will follow the local geometry of the interferometer. The Figure 5 shows schematically how a typical MBI result looks like in the SFA. The fringes are curved. Each fringe corresponds to a specific chromatic order N. Therefore, those fringes are called Fringes of Equal Chromatic Order (FECO).
More information about the following advanced topics about FECO's are also available on the web
- assymetric MBI with topography.
- reflection on inner boundaries.
- secondary fringes and their meaning.
- the simulation of FECO's